MONTE, Guidobaldo, Marchese Del.

Problematum Astronomicorum Libri Septem.

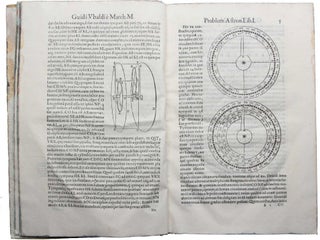
Venice: Bernardo Giunta & G.B. Ciotti, 1609.

First edition, very rare, of the principal astronomical work of one of the greatest Renaissance mathematicians. “Guidobaldo was Galileo’s patron and friend for twenty years and was possibly the greatest single influence on the mechanics of Galileo” (DSB). Guidobaldo was also influential in securing appointments for Galileo, first at Pisa and then Padua. Together they conducted a series of experiments on the trajectories of cannonballs, asserting that projectiles follow parabolic paths. The present work, originally composed in the 1580s but published posthumously by Guidobaldo’s son Orazio, is devoted to mathematical astronomy, but is particularly notable for its descriptions of several newly invented instruments, both for making astronomical observations and for carrying out the calculations involved in solving problems in astronomy. These include an ‘astronomical theodolite’ for measuring the altitude and azimuth of celestial bodies, and a geared instrument which can divide circular arcs into degrees, minutes, seconds, thirds, and so on. “Guidobaldo helped to develop a number of mathematical instruments, including the proportional compass, the elliptical compass, and a device for dividing the circle into degrees, minutes, and seconds” (DSB). “In general Guidobaldo’s attitude to mathematical instruments paralleled his attitude towards machines. Through these material devices, he felt, abstract mathematical truth could be made completely visible” (Rose, p. 224). A letter written by Orazio on 26 August 1609 documents that he “had contacted Galileo in order to ask advice about the publication [of the Problematum Astronomicorum]: Galileo seems to have answered to the former to have ‘learned many new things’ from the Problematum Astronomicorum Libri septem and to ‘appreciate it very much’” (Frank, p. 547). The first book of the Problematum Astronomicorum contains Guidobaldo’s descriptions of his instruments; the next four books deal with typical problems of mathematical positional astronomy; the sixth book is devoted to the estimation of the depression of the Sun towards the horizon at the beginning of the morning twilight and at the end of the evening twilight; and the seventh (and last) book is on comets. ABPC/RBH records no sales of this book at auction since the Macclesfield copy in 2005 (part of a sammelband), and only one before that (Christie’s New York, November 13, 1996, lot 408, $5,175).

Guidobaldo del Monte (1545-1607) was one of the most prominent Italian mathematicians from the second half of the sixteenth century. He was a nobleman from the Duchy of Urbino and occupied a central position at the courts of the Dukes Guidobaldo II and Francesco II della Rovere for large part of his life, until he fell out of favour in the 1590s. He studied mathematics at Padua, and later at Urbino he became the friend and pupil of Federico Commandino, whose translation of Pappus he edited and published. He combined practical work as an architect, designer of instruments, and surveyor of fortifications, with theoretical works on astronomy, perspective and mechanics. In cosmology he appears to have been an orthodox Aristotelian, as judged by his reaction to the supernova of 1604. Notwithstanding this latter position, he was also one of the most important patrons of the young Galileo. Together with his brother, Cardinal Francesco Maria del Monte (well known for having been a patron of Caravaggio), he helped Galileo secure his first teaching positions at the University of Pisa (in 1589) and Padua (in 1592). His “first book, the ‘Liber mechanicorum’ (1577), was regarded by contemporaries as the greatest work on statics since the Greeks [and his ‘Perspectivae libri sex’ (1600)] the best Renaissance study of perspective … Guidobaldo was Galileo’s patron and friend for twenty years and was possibly the greatest single influence on the mechanics of Galileo. In addition to giving Galileo advice on statics, Guidobaldo discussed projectile motion with him, and both scientists reportedly conducted experiments together on the trajectories of cannonballs. In Guidobaldo’s notebook (Paris MS 10246), written before 1607, it is asserted that projectiles follow parabolic paths; that this path is similar to the inverted parabola (actually a catenary) which is formed by the slack of a rope held horizontally; and that an inked ball that is rolled sideways over a near perpendicular plane will mark out such a parabola. Remarkably the same two examples are cited by Galileo at the end of the Two New Sciences, although only as postscripts to his main proof—which is based on the law of free fall—of the parabolic trajectory” (DSB).

“At the beginning of the Problematum astronomicorum (ff. 2v-3v), Guidobaldo, after having tackled the problem of graduated scales, proposes an instrument (ff. 7r-7v) to detect the height and azimuth of celestial bodies, that is a sort of astronomical theodolite … The Guidobaldo apparatus consists of two graduated metal circles: one horizontal with compass, the other vertical, the latter equipped with an alidade with open slit sights. The graduated circles present the engravings of the scales as in the more traditional astrolabes, to which the instrument appears closely related. The apparatus made it possible to sight angles of any width both for topographical and geographic surveys and for astronomical observations. Especially for the latter, an aspect of central importance was the appreciation of the fractions of a degree. Guidobaldo, aware of the extent of the problem, confronts it at the very beginning of the Problematum astronomicorum. He does so from a very general point of view, that is, he deals with reading on a standard scale, regardless of the instrument that hosts it: and then he tries to generalize it. What he describes is a recursive procedure that allows one in theory to go back not only to the usual fractions of a degree, that is to the first and second, but to proceed further, to infinity, that is to the ‘thirds,’ ‘quarters,’ ‘fifths,’ etc., where for example the ‘third’ is the sixtieth part of the second. Convinced of the feasibility of the method, Guidobaldo also perfects a mechanical tool for calculating fractions of degrees [see below] … In this regard, his observation on the dimensions of the graduated circles is significant – he estimates that a diameter of 1 foot (34 cm – the Pesaro foot) is sufficient to trace any fraction of a degree. This conviction led him to remain within the scope of tradition by proposing instruments of limited size, as opposed to the path taken by Tycho Brahe of constructing larger and larger instruments. From Guidobaldo’s point of view, the system of fractions of degree, not requiring large scales, was advantageous, as it avoided the design and construction problems typical of large instruments (weight, load on pins and supports, deformations, etc.)” (Gamba & Mantovani, pp. 220-222).

The new instrument Guidobaldo designed to calculate fractions of degrees is described on ff. 2v-6v of the Problematum astronomicorum. “It is a mechanical device that automatically multiplies by 60 not only the fractions of a degree, but also the first, second, ‘thirds,’ ‘quarters,’ etc. The problem, explains Guidobaldo, can be solved geometrically in a preliminary way using a normal compass. For example, if the alidade targets an angle between 26° and 27°, the procedure to be used is simple: with the tips of the compass, the fraction of a degree exceeding 26° is taken on the graduated scale, then, starting from the full scale, it is brought back to the same scale 60 times. The division of the scale on which it falls will indicate the desired fraction; if exact alignment with a division is not obtained, the fraction of a division thus obtained is again brought back to full scale with the compass and the procedure described above is repeated, obtaining the seconds, and so on, with an iterative process, until intercepting exactly one division. Guidobaldo performs these operations all on the same scale with graduation from 0° to 60°. The method, in addition to the expenditure of time, poses the problem of the precision and accuracy of the iterated measurements. To remedy this, Guidobaldo, inspired by watchmaking, proposes a rapid mechanical device, which in his opinion is precise, which automatically multiplies by 60 (ff. 5r-6v). It is a system of four gears that corresponds to a rotation of an index on a quadrant, 60 rotations of another index on an opposite quadrant. Consequently, a rotation of an arc of 1° corresponds, on the other quadrant, to a rotation of 60°” (ibid., p. 224).

Guidobaldo seems to have had the idea for this instrument from his friend, the Venetian patrician Jacomo Contarini (1536-95). “According to Muzio Oddi – an Urbino mathematician and pupil of Guidobaldo – Commandino in 1568 had designed a reduction compass at the request of Bartolomeo Eustachio. Soon afterwards Guidobaldo elaborated it into a proportional compass (or sector). [Contarini] was very much interested in mathematical instruments and he consulted Guidobaldo on the operation of a reduction compass related to Commandino’s, but invented some time before by one Fabrizio Mordente (d. 1608). A letter written by Guidobaldo on 6 October 1577 duly explained the operation of Mordent’s instrument, and it may also be the case that Guidobaldo transmitted its design as well to Contarini, for in a folio notebook of the latter’s there appear drawings and a description of such an instrument. Contarini’s notebook also contains two unpublished letters addressed to him by Guidobaldo in 1580 which refer to another instrument of mutual interest. This is a geared instrument which can divide circular arcs into degrees, minutes, seconds, thirds, and so on. Apparently Contarini had sent Guidobaldo such a device on which the marchese had modelled his own. He now asks Contarini’s permission to describe the mechanism in his forthcoming Problematum astronomicorum. In this work, there are instructions and diagrams relating to the instrument although Contarini’s name is not mentioned” (Rose, pp. 223-224).

Riccardi I 180; Houzeau & Lancaster 2912. Frank, Guidobaldo dal Monte’s Mechanics in Context, PhD thesis, University of Pisa, 2011. Gamba & Mantovani, ‘Gli strumenti scientifici di Guidobaldo del Monte,’ Chapter 10 in Guidobaldo del Monte (1545-1607), Becchi, Meli & Gamba (eds.), 2013.

Folio (232 x 210 mm), ff. [vi], 128 (numerous misfoliations but fully complete), with large engraved celestial sphere on title and hundreds of woodcut diagrams in text (slight water stain on outer margin of title and the next 12 leaves). Contemporary stiff vellum. A fine copy.

Item #2745

Price: $18,500.00