De resolutione et compositione mathematica libri quinque. Opus posthumum.
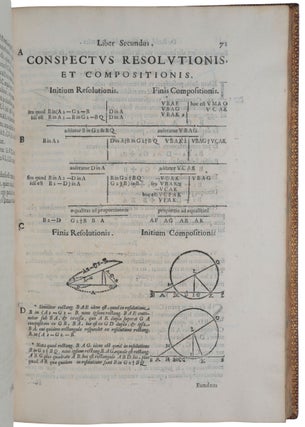
Rome: Camera Apostolica, 1630. First edition, extremely rare, and a magnificent copy from the library of Kenelm Digby, of the most important work of the great Croatian mathematician Marino Ghetaldi (or Marin Getaldić) who, while he did not introduce coordinates, is regarded by many scholars, on the basis of the present work, as the founder of analytic geometry – Descartes’ Géométrie was not published until seven years later. De resolutione is the first comprehensive work devoted to the application of the methods of symbolic algebra introduced in François Viète’s epoch-making In artem analyticam isagoge (1591) (a work of only 9 pages). “The final stage in the embryonic evolution of analytic geometry was to transfer the purpose of Viète’s analysis away from geometrical constructions to the solution of algebraic equations, and towards the application of the already well-known algebraic techniques to the solution of geometrical problems. This transformation was first effected by Viète’s contemporary and one-time pupil Marino Ghetaldi, in his posthumously published De resolutione et compositione mathematica (Rome, 1630). Here, the status of symbolic algebra was raised from a ‘means to an end’ to a method in its own right, with a wider scope and application than it had hitherto possessed … Ghetaldi has been hailed by various mathematicians as the father of analytic geometry … perhaps an acceptable compromise is to regard this science as having been conceived jointly by both of them … One might tentatively date the moment of conception as Ghetaldi’s first meetings with Viète in Paris, and the time of birth as shortly before [Ghetaldi’s] death when he is known to have composed this treatise … two things follow from the proposed interpretation of the birth of analytic geometry: one is that it did not require the adoption of the coordinate principle; and the other is that it occurred prior to the publication of Descartes’ Géométrie” (Forbes, pp. 147-8). “Getaldić’s mathematical works can be divided into two essentially different groups. The first group consists of the five works published while he was alive, the second consists of the posthumously published work [De resolutione]. In the first group of works, Getaldić solved geometrical problems by Greek methods, while in the last mentioned work he used algebraic analysis” (Dadić 1984, p. 208). De resolutione is divided into five books, the last being the most important. The third chapter in the fifth book deals with algebraic problems with infinitely many solutions, which Ghetaldi calls ‘Problemata Vana et Nugatoria’. Of these, the solution to the fourth problem is represented by a point which traces out a hyperbola when a certain length is varied; in the fifth problem a similar construction leads to an ellipse. These are regarded as the first descriptions of curves by means of algebraic equations. “Due to the fact that Getaldić came very close to the realization that all points that satisfy an indeterminate problem are on some curve, … [it] was argued that Getaldić, through his major work, indirectly participated in the preparation and creation of the synthesis of the arithmetic continuum of numbers and the geometric continuum of points, realized in Descartes’ analytical geometry, on the basis of which infinitesimal analysis later developed” (Borić, pp. 69-70). Ghetaldi met and corresponded with the Jesuit mathematicians Christopher Clavius, Christopher Grienberger and Paul Guldin, and also with Galileo, whose influence can be seen in Ghetaldi’s use in the present work of the terms analysis (resolutio) and synthesis (compositio), rather than Viète’s exegetics, poristics and zetetics. ABPC/RBH list only the Macclesfield copy in the last 35 years, and only one copy in the quarter-century before that. OCLC lists Brown, Columbia, Huntington, Kansas, Temple, and Yale in the US. Provenance: Sir Kenelm Digby (1603-65), philosopher (gilt arms on covers and his wife Venetia’s cypher ‘KVD’ on spine). The arms are the second of the four types listed on British Armorial Bindings (armorial.library.utoronto.ca/stamp-owners/DIG002). “Digby’s books are often readily recognisable from the various manifestations of his arms or monogram found on the boards or spine; he used 4 versions of his arms, two monogram stamps (one KD, one KDV adding V for his wife Venetia), and a fleur-de-lys in two sizes” (bookowners.online/Kenelm_Digby_1603-1665). Ghetaldi (1568-1626) was born to an old aristocratic Dubrovnik family originally from Taranto, Italy. After graduating from the Gymnasium, probably in 1588, he held various public positions, but found the atmosphere in Dubrovnik not conducive to his mathematical studies. He therefore embarked on a tour of Western Europe, arriving in Rome some time between 1594 and 1598, where he met Clavius and Grienberger. After two years spent in England, he arrived in Paris in February 1600, where he met and began his collaboration with Viète. As Ghetaldi wrote in a letter to the Belgian mathematician Michel Coignet, it was Viète who was most responsible for his development as a mathematician. Viètein turn must have appreciated Ghetaldi, both as a mathematician and as a friend, because he gave him transcripts of some of his unpublished works, and even allowed him to edit his work De numerosa potestatum resolutione (Paris, 1600). In 1600, Ghetaldi moved to Padua, where he became a member of Galileo’s circle; he became known to its members as the “demon of mathematics”. Here he was particularly influenced by Giovanni Vincentio Pinelli and Paolo Sarpi. In October 1602, Ghetaldi was back in Rome, where he associated with Luca Valerio, but in 1603 he was forced to return to Dubrovnik, with a ban on returning to Rome (probably because of a duel). Ghetaldi’s first two published works, which appeared at Rome in 1603, were Promotus Archimedes seu de variis corporum generibus gravitate et magnitudine comparatis, which deals with problems of specific gravity, and Nonnullae propositiones de parabola, a study of parabolic mirrors. Inspired by his meeting with Viète, Ghetaldi then turned to the task of reconstructing the lost works of Apollonius, which Viète himself had treated in his Apollonius Gallus (1600) – this led to two works published at Venice in 1607, Apollonius redivivus seu restituta Apollonii Pergaei inclinationum geometria (of which a second part appeared in 1613), and Supplementum Apollonii Galli seu exsuscitata Apollonii Pergaei tactionum geometriae pars reliqua. According to Dadić (1996, pp. 117-8), “Getaldić must certainly have employed algebraic analysis to solve the problems in the restored works of Apollonius,” although he presented his solutions in the classical Greek synthetic form. “Getaldić wrote to Guldin that he found difficult synthetic solutions by means of algebraic analysis … In his reply [of February 24, 1617], Guldin encouraged Getaldić, referring to his De resolutione et compositione mathematica, ‘to open the sources whence so many proofs, true cases, determinations, and deductions have flowed, which we have admired so much in the second book of Apollonius revived.’ Guldin also wrote that Getaldić had promised him ‘to describe in detail the technique, the method, and the way to do it in a book on mathematical analysis and synthesis.’ One may conclude that Getaldić deliberately presented the restorations of Apollonius’s works in the synthetic form regardless of whether he used the geometric or algebraic analysis while solving problems” (ibid., p. 118). In the third work Ghetaldi published in 1607, Variorum problematum collectio, he solved 42 geometrical problems. “He conceived Variorum problematum collectio and De resolutione et compositione mathematica at the same time. The problems he solved here [in the Variorum] were solved again in De resolutione. That he occupied himself with both works simultaneously is shown in the preface to his collection where it is said that he would solve some problems algebraically in De resolutione. In Variorum problematum collectio, Getaldić set forth the solutions he found by procedures that were not part of Viète’s algebraic method, and in his De resolutione he wanted to show the possibilities of the analytic-synthetic method” (ibid., p. 121). “Two kinds of analysis were distinguished in early modern geometry: the classical and the algebraic. The former method was known from examples in classical mathematical texts in which the constructions of problems were preceded by an argument referred to as ‘analysis;’ in those cases the constructions were called ‘synthesis’” (Bos, p. 95). The algebraic analysis used by Ghetaldi in De resolutione is more complicated, consisting of four distinct parts (ibid., p. 106): A: The derivation of an algebraic equation from the problem. A': The algebraic solution of the equation, or, if this is not possible, a rewriting of the equation in some standard form. B: The expression of the algebraic solution from A or A’ in words – this was called the ‘porism’. C: The solution of the problem in the form of a construction based on the porism. D: The proof that that construction was correct. Parts A and A' constituted the ‘analysis,’ part C the ‘synthesis’. The construction started from the given elements and operated on them in the manner indicated by the explicit expression in A or by the structure of the coefficients in the equation found in A'. Thus the direction of the synthesis was opposite to that of the analysis, but it did not retrace the steps of the analysis. In the case of the proof, part D, the elements of the analysis did recur, but not necessarily in their original order, and in combination with arguments from the construction. The first four books of De resolutione are devoted to algebraic and geometrical treatments of problems which, when put into equation form, do not exceed the second degree. The most complex problem treated here is Problem III of the fourth book (pp. 264-297), in which Ghetaldi proposes a method for determining the radius of the Earth. Widely differing estimates had been given by Archimedes, Hipparchus, Ptolemy, and others. Ghetaldi describes his method as follows: “Choose on the surface of a lake, when there is no wind, two positions at least three miles apart. At one position, a tin plate with a narrow opening extended in width should be raised perpendicular to the surface of the water. A light should be placed on the back of the plate at the opening, and the distance from the water surface to the top of the opening should be about one foot. At the other position, an observer raises or lowers his eye until the light from the plate reaches him in a direction that touches the surface of the water, so that if he lowers his eye he would no longer see the light due to the roundness of the surface of the water that would now come between the eye and the light. Now carefully measure the distance between the observer’s eye and the surface of the water, as well as the distance between the surface of the water and the top of the opening on the plate. From these distances, the diameter of the Earth can be calculated” (pp. 263-4, our translation). In mathematical terms, the problem is to determine the height of a triangle, given the length of its base and the amounts by which the lengths of the other two sides exceed the height. The method is sound, although it seems doubtful whether Ghetaldi actually carried out the necessary measurements. The first chapter of the fifth book deals with two very distinct topics: the application of algebraic analysis in the determination of specific gravities, especially the ‘Crown Problem’ of Archimedes; and a study of arithmetic progressions – how to find all the terms of the progression, when their sum, the first and the last term are given (Ghetaldi solves this problem using proportions). The second chapter treats nine ‘impossible’ problems, by which Ghetaldi means those which lead to equations with only imaginary roots or with zero as a root, which are incapable of geometrical interpretation. The third chapter treats five problems which have infinitely many solutions. In the first type, any value of the unknown is a solution, because the problem reduces to an equation which is an identity. The second type includes the fourth problem (p. 326), which we referred to earlier: to construct a triangle over a given base line, the other two legs of which have half the base line as their difference. Ghetaldi’s solution, for which he gives both an algebraic and a geometric proof, is as follows (see Bonifačić). Let AB be the base of the required triangle, let C be the mid-point of AB, let D be any point on the base, and let E be the mid-point of DB. Let F be the point on AB produced with AF = 2AD, and let H be the mid-point of CF. With centre A, draw an arc of a circle with radius AH, and let G be its intersection with the peropendicular to AB passing through E. Then ABG is the required triangle. Since the point D can be chosen arbitrarily on the base, there are infinitely many such triangles. In fact, the point G can be any point satisfying the equation CE2 – ⅓EG2 = AB2/16. Geometrically, this equation means that the vertex of the required triangle can be any point on a certain hyperbola. The fourth chapter contains eight problems which Ghetaldi was unable to reduce to algebra. For example, in Problem I (p. 330), a rhombus ABCD is given; a point K on AB produced must be determined so that, if the line KD cuts the side BC of the rhombus at the point I, the length of IK is a given value. The unknown length x of the extension BK then satisfies an equation of degree 4, which is presumably why Ghetaldi did not give an algebraic solution. He did not notice, as Descartes did when he treated the problem in La Géométrie, that this fourth degree equation factorises into the product of two quadratic equations, which enabled him to give a geometric construction for the solution. De resolutione was seen through the press by Ghetaldi’s youngest brother Jakova and his friend Ignjata Tudiševića. It was dedicated by the printer to Taddeo Barberini, the brother of Cardinal Francesco Barberini. The Barberini coat of arms, with three golden bees on an azure ground, featured prominently in the 17th century on various buildings, sculptures and other artefacts. The Barberini had earlier been the family of Tafani or horseflies, and had adopted the name of their town – along with a more attractive insect – as an aid to and index of their social rise. Bees were symbolic of moral virtue – they appeared to work extremely hard and produced something very desirable, they were hymned in the Bible, where their honey was compared to the Word of God, and bees also produced wax for candles, and were thus supportive of the Church. “Philosopher, alchemist and privateer, Kenelm Digby cut a striking figure across Europe in the middle of the 17th century. The son of a traitor executed for his part in the Gunpowder Plot, Digby was ‘more than two yards high’, a red-haired giant who spoke six or seven languages, and married the most famous beauty of his age, Venetia Stanley. John Aubrey said that he was ‘a goodly handsome person, gigantique and great voiced; had he been drop’t out of the clouds in any part of the world, he would have made himself respected.’ Digby turned from Catholicism to Anglicanism and back again, with as much verve as he turned from ecumenical theology to mechanical philosophy and then spurious medical recipes. He gained a huge reputation as a duelist and naval commander, counted Thomas Hobbes, Thomas White, The Earl of Dorset, Ben Jonson, and the painter Van Dyck amongst his good friends, and corresponded or conversed with Galileo, Descartes, Gilbert and Harvey; in his last years he was one of the founding members of the Royal Society” (Macdonald, p. 5). Van Dyck’s portrait of Digby is in the National Gallery in London. Lady Digby died in 1633, perhaps as a sad consequence of Digby’s amateur pharmacology, and he retired to Gresham College, where he occupied himself with chemical experiments for two years. After 1635 Digby associated himself with the entourage of Henrietta Maria, Charles I’s Catholic queen. In 1641 he went to France. “Shortly before his move to Paris, Digby reconverted back to Roman Catholicism, and upon his arrival at the French capital, became involved with another English ex-patriot, Thomas Hobbes, in the circle around Marin Mersenne. Through his entrée into Mersenne’s circle Digby quickly came to learn about Descartes’ work … In February 1640, Digby went to great lengths to obtain a private interview with Descartes at his retreat in Egmont, Holland; a letter from Digby to Descartes in March 1640 thanks him for their meeting” (MacDonald, p. 8). “Digby returned to London from Paris in 1642, to support Charles I in the hope of winning tolerance for Catholics, and was arrested twice and imprisoned – once for only a week, and the second time for almost a year (November 1642 to July 1643) … During his second period in captivity, Digby drafted the book that eventually appeared, in 1644, as Two Treatises: of Bodies and of Man’s Soul” (Clarke, p. 281). This was “the first comprehensive, systematic work of philosophy in the English language – something that is not well recognized at all. In this huge work he discussed at length every major theme we would recognize today as falling under the study of philosophy: elements, matter, mechanism, motion and causation, as well as sensation, perception, memory, imagination, intellect, reason, and immortality. Digby’s Two Treatises ‘shares with Descartes’ Principles of Philosophy the honour of being the first fully considered attempt at a mechanistic system” (Macdonald, p. 5). “Digby was one of the first (if not the first) to introduce Descartes to English readers; he was an early champion of Galileo and helped to bring the Italian’s ideas about mechanism, motion, and the heliocentric system to a wider English audience” (ibid., p. 10). In 1644 Digby again traveled to Paris, which remained his principal residence for a decade. Following the establishment of The Protectorate under Oliver Cromwell, Digby was received by the government as a sort of unofficial representative of English Roman Catholics, and was sent in 1655 on a mission to the Papacy to try to reach an understanding, but this proved unsuccessful. At the Restoration, Digby found himself in favour with the new regime due to his ties with Henrietta Maria, the Queen Mother. However, he was often in trouble with Charles II, and was once even banished from Court. Nonetheless, he was generally highly regarded until his death at the age of 62 from ‘the stone’. “Digby had an active interest in books, from both a scholarly and bibliophilic perspective, throughout his life and he assembled extensive collections. His correspondence, which survives in various places (including BL Add. mss 38175, 48146) includes numerous references to books and their acquisition. The library acquired up to the time of the Civil War, which was augmented by the bequest of Thomas Allen’s books, was apparently dispersed and possibly destroyed some time after 1643. Thereafter he assembled a library in Paris, which was left there on his return to England in 1660; he intended to return to it, but his death intervened. The collection, estimated at ca. 3500 volumes, was claimed as French crown property and mostly sold in Paris; Digby’s cousin George, 2nd Earl of Bristol (1612-77) purchased much of it and brought it back to England, where it was subsequently auctioned, along with the Earl’s own books and ‘the library of another learned person’ in London, 19 April 1680 … Although the catalogue states that ‘the great part … were the curiosities collected by the learned Sr. Kenelme Digby’, it is not possible to separate the books out with certainty … A manuscript catalogue of Digby’s French library, now in the Bibliothèque Nationale, shows that it was wide ranging in subjects and languages” (bookowners.online/Kenelm_Digby_1603-1665). The present volume is listed on page 10 of the printed catalogue of the sale of Digby’s second, ‘French,’ library, a copy of which is held by the Bibliothèque Nationale (NUMM-8711760). It was apparently not one of the books returned to England by Digby’s cousin, as it does not feature in the sale catalogue of his books, Bibliotheca Digbeiana, sive, Catalogus librorum in variis linguis editorum quos post Kenelmum Digbeium eruditiss. virum possedit illustrissimus Georgius Comes Bristol nuper defunctus : accedit & alia bibliotheca non minus copiosa & elegans : horum auctio habebitur Londini ... Aprilis 19, 1680 (http://name.umdl.umich.edu/A35964.0001.001). DSB V: 381-383; Poggendorff I, 890; Riccardi, 594, 6. Borić, ‘Marin Getaldić – a look on a new era. On occasion of the 450th anniversary of his birth,’ Croatian Studies Review 14-15 (2018/19), pp. 47-73. Bonifačić, ‘Gdje se Marin Getaldić najviše približio Descartesu,’ Anali Zavoda za povijesne znanosti Hrvatske akademije znanosti I umjetnosti u Dubrovniku 15-16 (1978), pp. 69-85; Bos, Redefining geometrical exactness, 2001. Clarke, Descartes, 2006. Dadić, ‘Some methodological aspects of Getaldić’s mathematical works,’ Historia Mathematica 11 (1984), pp. 207-214. Dadić, ‘The early geometrical work of Marin Getaldić,’ pp. 115-123 in: Vita Mathematica (Calinger, ed.), 1996. Forbes, ‘Descartes and the birth of analytic geometry,’ Historia Mathematica 4 (1977), pp. 141-151. Macdonald, Kenelm Digby’s Two Treatises, 2013.
Folio (320 x 220 mm), pp. [xii], 343, [1, blank], engraved Barberini arms on title and large woodcut bee device on *2v, woodcut diagrams in text. Contemporary French red morocco, covers ruled in gilt and with gilt arms of Sir Kenelm Digby, spine richly gilt in compartments with gilt lettering and cypher ‘KVD’ of Digby’s wife, Venetia, in each compartment, inner gilt dentelles, marbled endpapers, armorial bookplate (unidentified) featuring a double-headed eagle on front paste-down. A very fine copy.
Item #5465
Price: $65,000.00