De maximis et minimis geometrica divinatio in quintum Conicorum Apollonii Pergaei adhuc desideratum.
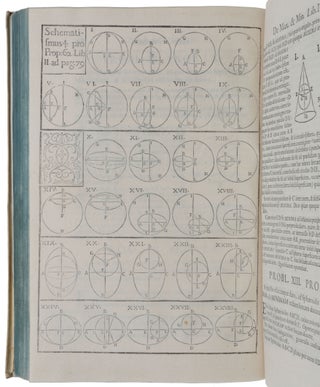
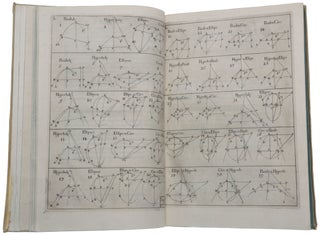
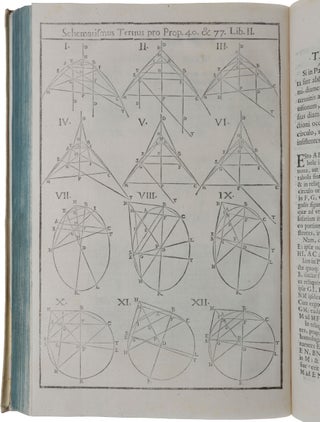
Florence: G. Cocchini, 1659. First edition, and a fine copy, of Viviani’s reconstruction of the fifth book of Apollonius of Perga’s Conics, “one of the greatest scientific books of antiquity” (Stillwell), alongside those of Euclid and Archimedes. Of the eight books of the Conics only the first four were known in Latin until the mid-17th century. An Arabic manuscript of three of the missing four books came to light while Viviani was working on his reconstruction; this was published by Borelli two years later. The consonance between Viviani’s reconstruction and Borelli’s edition provoked great admiration. “Apollonius’s theory of the conic sections (about 220 BC) is undoubtedly one of the masterpieces of ancient mathematics and will remain one of the great classics of mathematical literature” (Neugebauer, p. 136). To understand its importance in modern science, we need only recall Kepler’s use of the Conics in his Astronomia Nova, where it was fundamental for his proof of the ellipticity of the orbit of Mars, and Galileo’s use of Apollonius’ work when describing the parabolic trajectories of prjectiles. Books I-IV were first published in 1537. Book V is concerned with the straight lines perpendicular to conics (their ‘normals’), and with their ‘evolutes’ (curves tangent to all the normals). It “is the most remarkable of the extant Books. It deals with normals to conics regarded as maximum and minimum straight lines drawn from particular points to the curve. Included in it are a series of propositions which, though worked out by the purest geometrical methods, actually lead immediately to the determination of the evolute of each of the three conics [ellipse, parabola and hyperbola]; that is to say, the Cartesian equations to the evolutes can be easily deduced from the results obtained by Apollonius. There can be no doubt that the book is almost wholly original, and it is a veritable tour de force” (Heath, pp. 158-9). An appendix to De maximis et minimis (pp. 143-154) treats what is now called the ‘Steiner problem’ – to find a point in a plane such that the sum of its distances from three given points is a minimum (there is, in fact, a unique such point). It was first proposed by Fermat to Torricelli, who solved the problem and passed it to his student Viviani, who in turn published his own and Torricelli’s solutions in the present work. “Apollonius (ca. 245-190 BC) was the last of the great Greek mathematicians, whose treatise on conic sections represents the final flowering of Greek mathematics” (Hutchinson’s DSB, p. 16). Apollonius synthesized the work of his predecessors as well as contributing new methods and techniques of his own. “Hipparchus and Ptolemy absorbed his work and improved on it. The result, the Ptolemaic system, is one of the most impressive monuments of ancient science (and certainly the longest-lived), and Apollonius’ work contributed some of its essential parts … Apollonius has, in a way, suffered from his own success: his treatise became canonical and eliminated its predecessors, so that we cannot judge by direct comparison its superiority to them in mathematical rigor, consistency and generality … It is hard to underestimate the effect of Apollonius on the brilliant French mathematicians of the seventeenth century, Descartes, Mersenne, Fermat, and even Desargues and Pascal, despite their very different approach. Newton’s notorious predilection for the study of conics, using Apollonian methods, was not a chance personal taste … It was not until Poncelet’s work in the early nineteenth century … revived the study of projective geometry that the relevance of much of Apollonius’ work to some basic modern theory was realized” (DSB I: 97-99). “Viviani (1622-1703) was the son of Jacopo di Michelangelo Viviani, a member of the noble Franchi family, and Maria Alamanno del Nente. He studied the humanities with the Jesuits and mathematics with Settimi, a friend of Galileo’s. His intelligence and ability led to his presentation in 1638 to Ferdinand II de’ Medici, grand duke of Tuscany. Ferdinand introduced him to Galileo, who was so impressed by his talent that he took him into his house at Arcetri as a collaborator in 1639. After Galileo’s death, Viviani wrote a historical account of his life and hoped to publish a complete edition of his works. The plan, however, could not be carried out because of opposition by the Church-a serious blow not only to Viviani’s reputation but even more to the progress of science in Italy. Since he was unable to purse the evolution of mathematical ideas that were developing during that period, Viviani turned his talent and inventiveness solely to the study and imitation of the ancients. “Although the Medici court gave him much work, Viviani studied the geometry of the ancients. His accomplishments brought him membership in the Accademia del Cimento, and in 1696 he became a member of the Royal Society of London. In 1699 he was elected one of the eight foreign members of the Académie des Sciences in Paris. He declined offers of high scientific positions from King John II Casimir of Poland and from Louis XIV” (DSB). “For seventeenth-century Italian mathematicians and philosophers, [the restoration of Book V of the Conics] was the type of work that was crucial to attaining natural knowledge. The humanistic activity of restoring and translating ancient texts was not uncommon in Renaissance Italy, and from the early sixteenth century, many mathematicians translated Greek texts seen as valuable to the mixed mathematical sciences. In the case of Apollonius, Italy’s leading thinkers became familiar with the first four books of his work in 1566, when the Tuscan mathematician Federico Commandino published a Latin translation … Galileo also used Apollonius’ work on conic sections in the Fourth Day of Two New Sciences when providing a geometrical demonstration of the motion of projectiles. There is no doubt then that by the mid-seventeenth century, Italy’s leading mathematicians were not only using Apollonius’ work to solve physical problems, but were also eager to restore and comment on the missing works. “Viviani’s efforts to restore Apollonius’ missing work, therefore, are important for our understanding of post-Galilean thought, as well as Viviani’s relationship with his colleagues and patrons, all of whom were interested in the same topic. Viviani had long been working on restoring Book V, a task that fitted well with his later published commentaries on Euclidean geometry and Aristaeus’ text on conics. For Viviani, this task was a crucial part of his natural philosophical activities, including his contributions to the Accademia del Cimento, since it helped the understanding and improvement of classical mathematical sources for their application in physical problems, such as the study of free falling bodies that he and Galileo undertook in Arcetri. As a measure of the perceived importance of this work in seventeenth-century Italian thought, Viviani was even quite happy to collaborate on this task with his rival inside the Tuscan Court, Giovanni Borelli. As two of Italy’s leading mathematicians, Viviani and Borelli rivalled each other for favouritism inside the Medici Court, the status and respect that came from being the Court’s First Mathematician, a post formerly held by Galileo, was considered valuable for one’s career. Nevertheless, that rivalry was pout aside when it came to the restoration of Apollonius’ work. “While Viviani was completing his Apollonian treatise, in June 1658, Borelli discovered in the Grand Duke’s [Fernando de’ Medici’s] library an Arabic manuscript actually containing a translation of the Conics. He immediately took it to Rome in order to work with Abramo Echellense, an Arabic scholar, on the translation of the text. During the months that followed, Borelli attempted to facilitate Echellense’s task by explaining the mathematical propositions to him. However, from July to September of that year, Borelli wrote to Leopoldo [de’ Medici, co-founder with his brother Fernando of the Accademia del Cimento] about the increasingly slow progress of the translation, and claimed to be finding many errors in the Arabic manuscript. During his stay in Rome while undertaking this task, Borelli also maintained a correspondence with Viviani. On 29 June 1658, Borelli wrote to Viviani telling him of his discovery of the missing books and the plans for translating them, despite the fact that this could attract attention away from Viviani’s work on the same topic. “According to Modestino del Gaizo, by the beginning of July the Grand Duke became aware of the possible clash of publication dates and granted Viviani permission to publish his De maximis et minimis first. In fact, del Gaizo notes that upon hearing of this news, Borelli wrote as follows to Viviani on 20 July, perhaps abiding by the Grand Duke’s decision: ‘you should go ahead and enjoy this advantage, which I doubt whether I can enjoy, although I have by me a very compendious treatise on the conics, all written out in my own hand.’ Meanwhile, according to Barbensi, Borelli must have been ordered to delay his own translation … “Viviani’s De maximis et minimis turned out to be an excellent prediction of Apollonius’ propositions and geometrical demonstrations of normal to conics. A. Natucci, the only historian to have noted the accuracy of Viviani’s restoration, even suggests that once the translation of Apollonius’ work was published under Borelli’s editorship, ‘it then became possible to ascertain the substantial similarity between the two works.’ Indeed, not only did Viviani manage to provide propositions similar to those in Apollonius’ original treatise, but the logical construction of the geometrical demonstrations also closely resembled the original text” (Boschiero, pp. 89-92). “The general problem solved in [Book V of the Conics] may be stated as follows. For a given conic section and a given point P: (1) to construct all minimum and/or maximum straight lines from P to the conic; (2) to determine how the distance between P and a variable point X on the conic changes as PX moves away from the position of minimum or maximum. Apollonius considers the topic of the book to be minima and maxima to conics, and not as normals to conics (the term by which Heath’s version usually refers to them, misleadingly). Apollonius does indeed prove (Props. 27-33) that the minimum or maximum line from a point on a conic is perpendicular to the tangent at that point (i.e., in modern terminology, is a normal), but this is clearly secondary for him. In this respect he differed from his predecessors, who, to judge from the preface to Book V, seem to have treated minima, in so far as they discussed them at all, together with tangents in the ‘elements of conics’, i.e., considered them primarily as normals” (Toomer, pp. xxxviii-xxxix). ‘Steiner’s problem’ is named after the Berlin mathematician Jakob Steiner (1796-1863) although, as noted above, it was first proposed by Pierre de Fermat (1607-65) to Evangelista Torricelli (1608-47). Viviani’s solution, given in the appendix to the present work, makes use of what is now known as ‘Viviani’s theorem’, that for a point inside an equilateral triangle, the sum of the perpendicular distances from the point to the three sides is a constant. If A, B, and C are the three given points, Torricelli stated that the required point P which is the same distance from A, B, and C is that point at which each of A, B, and C is seen at an angle of 120°. To prove this, Viviani constructed an auxiliary triangle whose sides pass through A, B, C and are perpendicular to PA, PB, PC, respectively. This auxiliary triangle is equilateral, and applying Viviani’s theorem solves the problem. Cinti 135; Riccardi, II, 625 (‘Raro e pregiato’). Boschiero, ‘Post-Galilean Thought and Experiment in Seventeenth-century Italy: The Life and Work of Vincenzio Viviani,’ History of Science 43 (2005), pp. 77-100. Heath, A History of Greek Mathematics, vol. II, 1921. Neugebauer, ‘The astronomical origin of the theory of conic sections,’ Proceedings of the American Philosophical Society 92 (1948), pp. 136-138. Toomer (ed.), Apollonius Conics Books V to VII, 1990.
Two parts in one volume, folio (289 x 193 mm), pp. [16], [15]4 [sic]; [4], 154, [2, errata], half-title, titles printed in red and black, woodcut geometrical diagrams in text, two copper-engraved plates on one folding sheet, two wood-engraved plates, printed correction slip pasted onto single line of text at O4, blue edges. Contemporary vellum with arms (Polish?) stamped on covers featuring two ravens with a ring in their beak, spine with manuscript title and ‘X’ at head (some discolouration and slight wear to covers). A fine copy.
Item #5468
Price: $14,500.00