CLAIRAUT, Alexis Claude.

Recherches sur les courbes à double courbure.

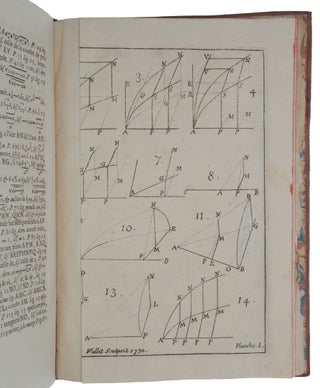
Paris: Nyon, Didot and Quillau, 1731.

First edition, very rare, of Clairaut’s first publication, the first extended treatment of the geometry of curves in three dimensions, written when he was only sixteen. In it Clairaut demonstrates a claim made by Newton that all cubic curves in three dimensions are projections of a cubic curve of a special form. This publication led to Clairaut’s election to the Académie des Sciences at the age of eighteen, the rules of admission being suspended to accommodate the mathematical prodigy. “Alexis Claude Clairaut was one of the most precocious of mathematicians, outdoing even Blaise Pascal in this respect. At the age of ten he was reading the textbooks of L’Hospital on conics and the calculus, when he was thirteen he read a paper on geometry to the Académie des Sciences ... In the year of his election Clairaut published a celebrated treatise, Recherches sur les courbesà double courbure, the substance of which had been presented to the Académie two years earlier ... The treatise of Clairaut carried out for space curves the program that Descartes had suggested almost a century before – their study through projections on two coordinate planes. It was, in fact, this method that suggested the name given by Clairaut to gauche or twisted curves inasmuch as their curvature is determined by the curvatures of the two projections. In the Recherches numerous space curves are determined through intersections of various surfaces, distance formulas for two and three dimensions are given, an intercept form of the plane is included, and tangent lines to space curves are found. This book by the teenage Clairaut constitutes the first treatise on solid analytic geometry” (Boyer, History of mathematics, p. 494). “Although the expression ‘double-curvature curve’ (gauche curve) is attributed to Henri Pitot (1724), Clairaut’s treatise on this type of figure is no less original, representing the first serious analytical study of it. The curve is determined by two equations among the three orthogonal coordinates of its ‘point courant’ (locus). Assimilation of the infinitesimal arc to a segment of a straight line permits determination of the tangent and the perpendiculars. It also permits rectification of the curve. This work also includes quadratures and the generation of some special gauche curves” (DSB). “In his 1731 work Recherches sur les courbes à double courbure, Clairaut studied tangents to curves of double curvature. He saw that a space curve can have an infinity of normals located in a plane perpendicular to the tangent. The expressions for the arc length of a space curve and the quadrature of certain areas on surfaces are also due to him” (Kline, Mathematical Thought from Ancient to Modern Times, p. 557). ABPC/RBH list only one other copy (Macclesfield).

Analytic geometry in two dimensions, i.e., the study of curves in the plane, dates from the work of Descartes, Fermat, Ghetaldi and others in the first half of the seventeenth century. Phillipe de la Hire understood, later in the century, that an equation in three unknowns represents a surface, but he did not study surfaces by means of their equations. John Wallis and Christopher Wren discussed the lines on the single-sheeted hyperboloid in the 1660s, but they did not use coordinates. Indeed, curves and surfaces in three dimensions were not studied in any detail until the eighteenth century.

The first explicit analytic treatment of a curved surface was given by Antoine Parent in 1700, “an awkward treatment of the surface of a sphere, but it shows a full knowledge of space coordinates” (Boyer, History of Analytic Geometry, pp. 156-157). However, this work seems to have made little impression upon his contemporaries. Jean Bernoulli, in correspondence with Leibniz in 1715, also showed familiarity with space coordinates, but this too long went unpublished and unnoticed. It was Euler, in a paper written in 1730 and published in the St. Petersburg Commentarii in 1732 (‘De linea brevissima in superficie quacunque duo quaelibet puncta iungente’), who presented for the first time a reasonably systematic treatment of whole classes of surfaces. Surprisingly, Euler did not consider space curves, but examples of these were studied by Henri Pitot: in the Paris Memoires for 1724, he studied the helix on a cylinder “and prophetically said that perhaps some day ‘curves of double curvature’, a name he coined for the first time, would be the object of researches in geometry … He did not carry such work further, however, and the systematic study of skew curves really began about half a dozen years later” (ibid., p. 166).

Clairaut’s “Recherches sur les courbes à double courbure was presented to the Académie des Sciences in 1729 when Clairaut was only sixteen years old, but it was published two years later. The Recherches, like the Géométrie of Descartes, appeared without the name of the author on the title page, although this was generally known. It carried out for space curves the program that Descartes had suggested almost a century before – their study by a consideration of the projections on two coordinate planes. From the preface of his book one gathers that he knew only of the work of Descartes, Bernoulli, and Pitot in three dimensions, and not of the far more important contributions of La Hire, Parent, and Euler. It was apparently a memoir by Jean Bernoulli which first drew his attention to the study of surfaces.

“One might have expected Clairaut to open with the straight line, or at least to consider first the theory of surfaces; but he began with a study of a space curve with reference to a single axis. He calls his coordinates x, y, z and says that if the z’s are connected with the x’s and with the y’s by non-linear equations, then they determine a space curve. He calls it a ‘curve of double curvature,’ following Pitot, because its curvature is determined by that of two curves obtained by projection of the original curve upon two perpendicular planes. Clairaut said that he would give only algebraic curves, but he asserted that transcendental curves are just as easily handled. He promised, in the preface, to give later a treatise on ‘curves given by coordinates from a point’ – i.e., polar coordinates in three dimensions. This would have been the earliest use of such a system, but unfortunately the work never appeared.

“Clairaut implicitly corrected the error of Descartes on normals by pointing out that such a curve has infinitely many normal lines; and he saw that, for a given space curve, any two projecting cylinders y = f(x) and z = g(y) determine a third z = F(x) = g(f(x)). This suggested the use of three mutually perpendicular coordinate planes instead of the single plane and axis with which he had begun.

“Clairaut’s discussion of surfaces is surprisingly similar to that of Euler, but less systematic. He first gave as examples the sphere a² = x² + y² + z², the cone z = √x² + y², and the paraboloid y² + z² = ax. Then he went on to give the equations of other surfaces of revolution, such as the ellipsoid, the one-sheeted hyperboloid, and the surface obtained by revolving the parabola ay = x² about the tangent at the vertex. Clairaut gave a treatment of general conical surfaces, using as generating curves the higher parabolas, ellipses, and hyperbolas. Like Euler, he knew that the equation of a cone with vertex at the origin is homogeneous. Clairaut also gave numerous examples of curves defined not by projecting cylinders but through the intersection of other surfaces; and he determined whether or not these curves lie on a given surface. He considered both positive and negative coordinates, although only portions of the figures are given in the diagrams. He showed how to sketch a surface by considering plane sections, using Bernoulli’s surface xyz = a³ as an illustration. He knew that the equation of a plane is linear in the variables … Clairaut included in book II some results on tangent and normal lines to a space curve, making use of the calculus; and the Recherches closes with book III in which the integral calculus is applied to curves and surfaces. This work is carried through in geometrical form dependent upon diagrams, indicating that the development of differential geometry waited upon further work by Euler and Monge.

“There is one point in plane coordinate geometry where Clairaut made an innovation of some interest. Historical accounts of analytical geometry place considerable emphasis upon the introduction of the distance formula, generally thought to have taken place in 1797 and 1798. However, the familiar formulae, for both two and three dimensions, are found in the Recherches of Clairaut. They are, of course, incidental to other matters, but they are clearly stated in connection with the determination of the equation of a spherical surface … Consequently, credit for them should go, pending further evidence, to Clairaut” (ibid., pp. 167-169).

Alexis Claude Clairaut was born in Paris to Jean-Babtiste Clairaut, who taught mathematics in Paris and was elected to the Berlin Academy. Jean-Baptiste Clairaut educated his son at home and set high standards, using Euclid’s Elements while learning to read. However, Alexis was a child prodigy – by the age of nine he had mastered the excellent mathematics textbook of Guisnée (Application de l’Algèbre a la Géométrie), which provided a good introduction to the differential and integral calculus as well as analytical geometry. He went on to study de L’Hôpital‘s books, in particular his famous text Analyse des infiniment petits pour l’intelligence des lignes courbes.

At the age of twelve Alexis wrote a memoir on four geometrical curves and under his father’s tutelage he made such rapid progress in the subject that in his thirteenth year he read before the Académie française an account of the properties of four curves which he had discovered. When only sixteen he finished his Recherches, which procured his admission into the French Academy of Sciences in 1729, but the king did not confirm his election until July 1731, when Clairaut became the youngest person ever elected to the Paris Academy of Sciences.

In the Academy, Clairaut became interested in geodesy through Cassini’s work on the measurement of the meridian. He allied himself with Pierre Louis Maupertuis, who became a close friend, and the small but youthful and pugnacious group supporting Newton. In 1736, together with Maupertuis, he took part in the expedition to Lapland, which was undertaken for the purpose of estimating a degree of the meridian arc. Maupertuis was director of the expedition, which included Le Monnier, Camus, the Abbé Outhier, and Celsius. The goal of the expedition was to geometrically calculate the shape of the Earth, which Issac Newton theorized in his book Principia was an oblate ellipsoid (fatter at the equator than at the poles). Cassini and his supporters believed it to be a prolate ellipsoid (thinner at the equator than at the poles).

After his return, Clairaut published his treatise Théorie de la figure de la terre (1743), in which he promulgated the theorem, known as Clairaut’s theorem, which connects the gravity at points on the surface of a rotating ellipsoid with the compression and the centrifugal force at the equator. Under the assumption that the Earth was composed of concentric ellipsoidal shells of uniform density, Clairaut’s theorem could be applied to it, and allowed the ellipticity of the Earth to be calculated from surface measurements of gravity. This proved Newton’s theory that the shape of the Earth was an oblate ellipsoid.

Clairaut guided the Marquise du Châtelet in her studies, especially in her translation of Newton’s Principia and in preparing the accompanying explanations, a project which began before 1745 and continued until part of the book was published in 1756.

4to (247 x 177 mm), pp. [8], 119, [3], with six folding engraved plates. Contemporary calf, spine gilt in compartments (a few surface scratches to the boards).

Item #5816

Price: $8,500.00