LAMBERT, Johann Heinrich.

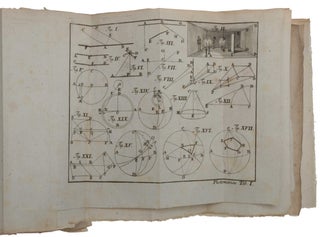
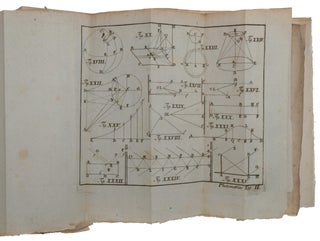
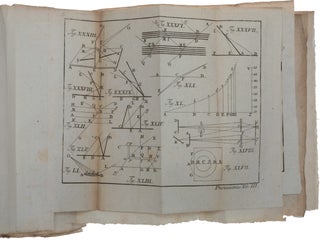
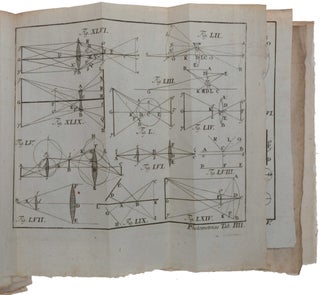
Photometria sive de mensura et gradibus luminis, colorum et umbrae.

Augsburg: Christoph Peter Detleffsen for the widow of Eberhard Klett, 1760.

First edition, very rare, and the finest copy we have seen, of the foundation work of photometry, the measurement of the intensity of light, both objectively and as perceived by the eye; this is one of the rarest of modern science books of this stature. Lambert’s discoveries “are of fundamental importance in astronomy, photography and visual research generally … Both Kepler and Huygens had investigated the intensity of light, and the first photometer had been constructed by Pierre Bouguer (1698-1758); but the foundation of the science of photometry – the exact scientific measurement of light – was laid by Lambert’s ‘Photometry’ … In the Photometria he described his photometer and propounded the law of the absorption of light named after him. He investigated the principles and properties of light, of light passing through transparent media, light reflected from opaque surfaces, physiological optics, the scattering of light passing through transparent media, the comparative luminosity of the heavenly bodies and the relative intensities of coloured lights and shadows” (PMM). “It is difficult to overstate how original most of Photometria was and how great was the advance Lambert made with it. He was the first to accurately identify most fundamental photometric concepts, to assemble them into a coherent system of photometric quantities, to define these quantities with a precision sufficient for mathematical statement, and to build from them a system of photometric principles … The behavior of point light sources had been understood since Kepler’s time. Lambert was the first to correctly solve the problem posed by extended light sources. He was the first to state the cosine emanation law, which describes how a surface of perfect diffusion emits light, and to see the far-reaching consequences of this idea. Lambert spent one tenth of Photometria developing equations for the calculation of illumination at points and surfaces from luminous areas of various forms – equations that are now used in modern computer graphics, and thermal and lighting engineering … With his emanation law, Lambert calculated the average brightness of the moon and planets, anticipating part of modern astrophysics by a century … Lambert made extensive measurements of the photometric properties of materials by ingenious application of the process of equating two brightnesses. He determined the reflectance and transmittance of glass for a range of angles, the brightness of images produced by lenses, the diffuse reflectance of matte surfaces, and the absorption of light in glass and in the atmosphere. He measured the color composition of white and colored surfaces and was the first to mix colored light and record that the result was different from mixing colored pigments … Lambert was the first to attempt to establish a relationship between the subjective assessment of a luminous stimulus – the brightness – made by the visual system, and the strength – the luminance and size – of that stimulus” (Dilaura, pp. i-ii). ABPC/RBH record the sale of three copies since Norman. OCLC lists copies in US at Brown, Harvard Medical School and Oklahoma.

Provenance: Georg Hermann Quincke (1834-1924) (signature on front free endpaper). Quincke received his Ph. D. in 1858 at Berlin, having previously studied also at Königsberg and at Heidelberg. He became privatdozent at Berlin in 1859, professor at Berlin in 1865, professor at Würzburg in 1872, and in 1875 was called to be professor of physics at Heidelberg, where he remained until his retirement in 1907.

Quincke did important work in the experimental study of the reflection of light, especially from metallic surfaces.

“Lambert began with two simple axioms: light travels in a straight line in a uniform medium and rays that cross do not interact. Like Kepler before him, he recognized that ‘laws’ of photometry are simply consequences and follow directly from these two assumptions. In this way Photometria demonstrated (rather than assumed) that

1. Illuminance varies inversely as the square of the distance from a point source of light.
2. Illuminance on a surface varies as the cosine of the incidence angle measured from the surface perpendicular.
3. Light decays exponentially in an absorbing medium.

“In addition, Lambert postulated a surface that emits light (either as a source or by reflection) in a way such that the density of emitted light (luminous intensity) varies as the cosine of the angle measured from the surface perpendicular. In the case of a reflecting surface, this form of emission is assumed to be the case, regardless of the light's incident direction. Such surfaces are now referred to as ‘Perfectly Diffuse’ or ‘Lambertian’.

“Lambert demonstrated these principles in the only way available at the time: by contriving often ingenious optical arrangements that could make two immediately adjacent luminous fields appear equally bright (something that could only be determined by visual observation), when two physical quantities that produced the two fields were unequal by some specific amount (things that could be directly measured, such as angle or distance). In this way, Lambert quantified purely visual properties (such as luminous power, illumination, transparency, reflectivity) by relating them to physical parameters (such as distance, angle, radiant power, and color). Today, this is known as ‘visual photometry.’ Lambert was among the first to accompany experimental measurements with estimates of uncertainties based on a theory of errors and what he experimentally determined as the limits of visual assessment.

“Although previous workers had pronounced photometric laws 1 and 3, Lambert established the second and added the concept of perfectly diffuse surfaces. But more importantly, as Anding pointed out in his German translation of Photometria [Leipzig, 1892], ‘Lambert had incomparably clearer ideas about photometry’ and with them established a complete system of photometric quantities. Based on the three laws of photometry and the supposition of perfectly diffuse surfaces, Photometria developed and demonstrated the following:

1. Just noticeable differences. In the first section of Photometria, Lambert established and demonstrated the laws of photometry. He did this with visual photometry and to establish the uncertainties involved, described its approximate limits by determining how small a brightness difference the visual system could determine.

2. Reflectance and transmittance of glass and other common materials. Using visual photometry, Lambert presented the results of many experimental determinations of specular and diffuse reflectance, as well as the transmittance of panes of glass and lenses. Among the most ingenious experiments he conducted was that to determine the reflectance of the interior surface of a pane of glass.

3. Luminous radiative transfer between surface. Assuming diffuse surfaces and the three laws of photometry, Lambert used Calculus to find the transfer of light between surfaces of various sizes, shapes, and orientations. He originated the concept of the per-unit transfer of flux between surfaces and in Photometria showed the closed form for many double, triple, and quadruple integrals which gave the equations for many different geometric arrangements of surfaces. Today, these fundamental quantities are called View factors, Shape Factors, or Configuration Factors and are used in radiative heat transfer and in computer graphics.

4. Brightness and pupil size. Lambert measured his own pupil diameter by viewing it in a mirror. He measured the change in diameter as he viewed a larger or smaller part of a candle flame. This is the first known attempt to quantify pupillary light reflex.

5. Atmospheric refraction and absorption. Using the laws of photometry and a great deal of geometry, Lambert calculated the times and depths of twilight.

6. Astronomic photometry. Assuming that the planets had diffusely reflective surfaces, Lambert attempted to determine the amount of their reflectance, given their relative brightness and known distance from the sun. A century later, Zöllner studied Photometria and picked up where Lambert left off, and initiated the field of astrophysics.

7. Demonstration of additive color mixing and colorimetry. Lambert was the first to record the results of additive color mixing. By simultaneous transmission and reflection from a pane of glass, he superimposed the images of two different colored patches of paper and noted the resulting addtive color.

8. Daylighting calculations. Assuming the sky was a luminous dome, Lambert calculated the illumination by skylight through a window, and the light occluded and interreflected by walls and partitions.

“Lambert’s book is fundamentally experimental. The forty experiments described in Photometria were conducted by Lambert between 1755 and 1760, after he decided to write a treatise on light measurement. His interest in acquiring experimental data spanned several fields: optics, thermometry, pyrometry, hydrometry, and magnetics. This interest in experimental data and its analysis, so evident in Photometria, is also present in other articles and books Lambert produced. For his optics work, extremely limited equipment sufficed: a few panes of glass, convex and concave lenses, mirrors, prisms, paper and cardboard, pigments, candles and the means to measure distances and angles.

“Lambert’s book is also mathematical. Though he knew that the physical nature of light was unknown (it would be 150 years before the wave-particle duality was established) he was certain that light's interaction with materials and its effect on vision could be quantified. Mathematics was for Lambert not only indispensable for this quantification but also the indisputable sign of rigor. He used linear algebra and calculus extensively with a matter-of-fact confidence that was uncommon in optical works of the time. On this basis, Photometria is certainly uncharacteristic of mid-18th century works.

“Lambert began conducting photometric experiments in 1755 and by August 1757 had enough material to begin writing. From the references in Photometria and the catalogue of his library auctioned after his death, it is clear that Lambert consulted the optical works of Newton, Bouguer, Euler, Huygens, Smith, and Kästner. He finished Photometria in Augsburg in February 1760 and the printer had the book available by June 1760.

“Maria Jakobina Klett (1709–1795) was owner of Eberhard Klett Verlag, one of the most important Augsburg ‘Protestant publishers.’ She published many technical books, including Lambert’s Photometria, and 10 of his other works. Klett used Christoph Peter Detleffsen (1731–1774) to print Photometria. Its first and only printing was evidently small, and within 10 years copies were difficult to obtain. In Joseph Priestley's survey of optics of 1772 [The History and Present State of Discoveries relating to Vision, Light, and Colours], ‘Lambert’s Photometrie’ appears in the list of books not yet procured. Priestley makes a specific reference to Photometria; that it was an important book but unprocurable.

“Photometria presented significant advances and it was, perhaps, for that very reason that its appearance was greeted with general indifference. The central optical question in the middle of the 18th century was: what is the nature of light? Lambert work was not related to this issue at all and so Photometria received no immediate systematic evaluation, and was not incorporated into the mainstream of optical science. The first appraisal of Photometria appeared in 1776 in Georg Klügel’s German translation of Priestley’s 1772 survey of optics. An elaborate reworking and annotation appeared in 1777. Photometria was not seriously evaluated and utilized until nearly a century after its publication, when the science of astronomy and the commerce of gas lighting had need for photometry. Fifty years after that, Illuminating Engineering took up Lambert’s results as the basis for lighting calculations that accompanied the great expanse of lighting early in the 20th century. Fifty years after that, computer graphics took up Lambert’s results as the basis for radiosity calculations required to produce architectural renderings. Photometria had significant, though long delayed influence on technology and commerce once the industrial revolution was well underway, and is the reason that it was one of book listed in Printing and the Mind of Man” (David Dilaura in Wikipedia).

“Johann Heinrich Lambert was born on 26 August 1728 in Mühlhausen (today Mulhouse, France). Mühlhausen was at that time associated to Switzerland. Lambert received six years of formal education from the municipality but had to leave school to help his father, a tailor, when he was 12 years old. However, Lambert never stopped learning though he did not attend any formal school afterwards. He studied French, Latin and Mathematics largely on his own. He became an assistant to the city clerk of Mühlhausen, J. H. Reber, then a bookkeeper to an industrialist and finally in 1746 a secretary to Prof. J. R. Iselin in Basel. In this position he gained access to the knowledge of physics and mathematics of his time. In 1748 he obtained a position in Chur as a private tutor to a grandson of Count Peter von Salis. At the court of von Salis, Lambert could finally pursue his research on physics and optics. He travelled with his pupil through Europe, meeting many eminent scientists and continuously pursuing his research. He became a member of the ‘physikalisch-mathematische Gesellschaft’ of Basel in 1754. From 1759 Lambert travelled on his own through Europe. During that time he published his early masterpiece, the Photometria.

“Lambert was living in rather poor conditions, though he received some support from the academies he was a member of. After long deliberations and in spite of Lambert’s eccentric character he became a member of the Royal Academy of Berlin in 1765. Finally Lambert had a secure post and he started researching and publishing on diverse topics of his interest. In this time of high productivity he proved that π and e are irrational, wrote about philosophy, studied non-additive probabilities and made contributions to hyperbolic functions and to cartography. Lambert died in Berlin on 25 September 1777” (Hulliger, pp. 2-3).

Grolier/Horblit 62; Norman 1269; PMM 205. Dilaura, Photometry … Translation from the Latin of Photometria, 2001. Hulliger, ‘Johann Heinrich Lambert,’ Bulletin of the Swiss Statistical Society 14 (2003), pp. 4-10.

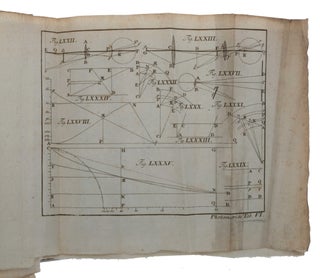
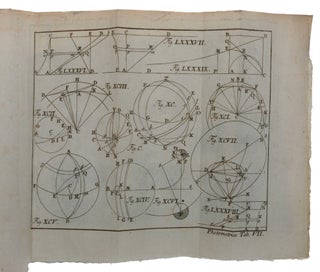
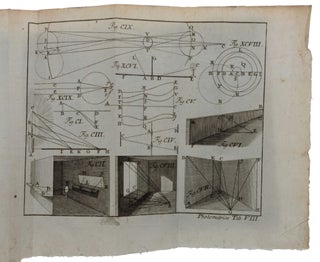
8vo (203 x 128 mm), pp. [xvi], 547, [13], with eight folding engraved plates, uncut. Contemporary marbled wrappers (a little rubbed). A fine copy with much less browning and spotting than is usually found with this book.

Item #5873

Price: $80,000.00