Traité de dynamique, dans lequel les loix de l'equilibre & du mouvement des corps sont réduites au plus petit nombre possible, & démonstrées d’une manière nouvelle, & où l’on donne un principe général pour trouver le mouvement de plusieurs corps qui agissent les uns sur les autres, d’une manière quelconque.
Paris: David l’ainé, 1743. First edition of one of the classics in the history of science, d’Alembert’s formalization of the new science of mechanics. “The ‘Treatise on Dynamics’ was d’Alembert’s first major book and it is a landmark in the history of mechanics. It reduces the laws of the motion of bodies to a law of equilibrium. Its statement that 'the internal forces of inertia must be equal and opposite to the forces that produce the acceleration' is still known as ‘d’Alembert’s principle’. This principle is applied to many phenomena and, in particular, to the theory of the motion of fluids. It has become useful in the practical solution of many technical and mechanical problems, and is as important for the motion of bodies as is the principle of virtual velocities for their equilibrium – the latter formulated by Johann Bernoulli in 1717. It was left to Lagrange to combine both these principles and to construct mechanical equations applicable to the motions of any system of bodies” (Printing and the Mind of Man, 195). “In the first part of the treatise Alembert developed his own three laws of motion: inertia, the parallelogram of motion, and equilibrium; his third law assumed conservation of momentum and defined mass accordingly. The second part contains the first statement of what is now known as ‘Alembert’s principle’ (Norman). “The Traité de dynamique, which has become the most famous of his scientific works, is significant in many ways. First, it is clear that d’Alembert recognized that a scientific revolution had occurred, and he thought that he was doing the job of formalizing the new science of mechanics … The Traité also contained the first statement of what is known as d’Alembert’s principle. D’Alembert was, furthermore, in the tradition that attempted to develop mechanics without using the notion of force. Finally, it was long afterward said (rather simplistically) that in this work he resolved the famous vis viva controversy (whether, in modern terms, it is momentum or kinetic energy that measures the ‘quantity of motion’), a statement with just enough truth in it to be plausible” (DSB). The second work, in which d’Alembert laid the foundations of scientific meteorology, is an application of the methods set out in the Traité de dynamique. He rejected the conception of Edmund Halley that the general circulation of the atmosphere is significantly controlled by the distribution of solar heating, and instead explained the origin of winds by means of the gravitational effects of the sun and moon. “The [Traité de dynamique] was published in 1743 by David, the great bookselling and printing house, … presented to the Académie des Sciences on 22 June and given a favourable review by the commissioners P. L. Maupertuis and F. Nicole. It includes a letter to Count de Maurepas, a 26-page preface summarizing and commenting on the main general ideas, and finally the body of the text with all its trimmings (table of contents, plates, corrections, extract from the records of the Académie des Sciences, royal favour). “Contrary to the author’s current custom, the book is clearly structured. Following the definitions and preliminary notions (pp. 1–2), the first Part is entitled ‘General laws of motion and equilibrium of bodies’ (pp. 3–48). It consists of three chapters, each of which is subdivided into articles numbered continuously. These chapters represent the three great principles on which dynamics is based: I. ‘On the force of inertia’ (arts. 2– 20); II. ‘On composite motion’ (arts. 21–26); III. ‘On motion destroyed or changed by obstacles’ (arts. 27–49). This last chapter contains, in particular, the theory of equilibrium. “The second Part, which is much larger (pp. 49–186), is entitled ‘A general principle for finding the motion of many bodies that act on each other in an arbitrary way, with many applications of this principle’. It consists of four chapters of disparate length and status: I. ‘Exposition of the principle’ (art. 50); II. ‘Properties of the centre of gravity of many bodies combined, deduced from the preceding principle’ (arts. 51–72); III. ‘Problems illustrating the application of the preceding principle’ (arts. 73–153); IV. ‘On the principle of conservation of live forces’ (arts. 154–175). What is today called ‘D’Alembert’s principle’ constitutes the single article of Chapter I. The rest of the second Part consists of what the author calls ‘applications’ … “‘D’Alembert’s principle’ (Chapter I of Part II) plays a pivotal role in the book: Part I paves the way for it and Chapters II–IV of Part II consist of applications. Moreover, it is this principle that posterity has universally accepted as one of D’Alembert’s main contributions to science” (Crépel, pp. 161-3). “It was not so much a principle as it was a rule for using the previously stated laws of motion. It can be summarized as follows: In any situation where an object is constrained from following its normal inertial motion, the resulting motion can be analyzed into two components. One of these is the motion the object actually takes, and the other is the motion “destroyed” by the constraints. The lost motion is balanced against either a fictional force or a motion lost by the constraining object. The latter case is the case of impact, and the result is the conservation of momentum (in some cases, the conservation of vis viva as well). In the former case, an infinite force must be assumed. Such, for example, would be the case of an object on an inclined. The normal motion would be vertically downward; this motion can be resolved into two others. One would be a component down the plane (the motion actually taken) and the other would be normal to the surface of the plane (the motion destroyed by the infinite resisting force of the plane)” (DSB). “D’Alembert deduces from [his principle] that the determination of all motions reduces to applying the principle of equilibrium and that of composite motion. That is why it is often said that D’Alembert’s principle reduces dynamics to statics. The simplest example is that of a body without elasticity obliquely striking a fixed impenetrable wall: the only component of motion preserved after the impact is that parallel to the wall, the component perpendicular to the wall being destroyed (Part I, Chapter III). A typical theorem from Chapter II is as follows: ‘The state of motion or rest of the centre of gravity of many bodies does not change under the mutual action of these bodies provided that the system is entirely free, that is, it is not subject to motion around a fixed point’. “Chapter III, which is by far the longest and takes up more than half of the book, contains a detailed treatment of 14 problems, divided into Sections as follows: I. ‘Bodies pulled by wires or rods’ (Problems I–VI); II. ‘Bodies moving in the plane’ (Problem VII); III. ‘Bodies acting on one another via wires along which they can run freely’ (Problem VIII); IV. ‘Bodies which move or collide’ (Problems IX–XIV). These problems are treated at unequal length, some being more famous than others. For example, Problem V (arts. 98– 112), on the period of oscillation of a composite pendulum, formed a part of the immediate prehistory of the problem of the vibrating string. “In Chapter IV, the author emphasizes the fact that, contrary to the Bernoullis, he does not assume the conservation of live forces, but that it can be deduced from his principle and methods. He states that he gives, ‘if not a general proof for all cases, at least a sufficient number of principles for finding the proof in each particular case’. He sketches these proofs for bodies on wires or rods in the case of elastic impacts, and for fluids” (Crépel, pp. 163-4). “A major question that beset all philosophers of the Enlightenment was that of the nature of matter … [D’Alembert] tended to accept the corpuscular theory of matter, and in Newton’s style; that is, he conceived of the ideal atom as perfectly hard. Since this kind of atom could not show the characteristic of elasticity, much less of other chemical or physical phenomena, he was sorely perplexed. In his Traité de dynamique, however, he evolved a model of the atom as a hard particle connected to its neighbors by springs. In this way, he could explain elasticity, but he never confused the model with reality” (DSB). “The Traité de dynamique permeates a large part of his work, quite explicitly so in the case of the Traité des fluides, which was published in the following year (1744) as a continuation of the earlier work. It is also true that the majority of his work on the physical sciences make use of D’Alembert’s principle, as do the Recherches sur la cause des vents of 1747 of those on the Précession des equinoxes two years later, or the Essai sur la résistance des fluides of 1752. It may be said that, even if these treatises are bursting with other interesting discoveries, they are also ‘applications’ of the treatise” (Crépel, p. 165). “A natural son of the chevalier Destouches and Mme. De Tencin, D’Alembert was born on 16 or 17 November 1717 and was placed (rather than abandoned) on the steps of the church of Saint-Jean-le-Rond in Paris—whence his given name, although much later he preferred ‘Daremberg’, then ‘Dalembert’ or ‘D’Alembert’. He followed his secondary studies at the Quatre-Nations College in Paris, and later studied law and probably a little medicine. His first memoir was submitted to the Académie des Sciences in Paris in 1739, and he became a member of that institution in 1741. The Traité de dynamique was D’Alembert’s first major work, to be followed by many others in the 1740s and early 1750s. He was co-editor with Dénis Diderot of the Encyclopédie, for which he wrote the introduction (1751) and around 1700 articles, mainly scientific, the majority of them before the work was banned following his article ‘Genève’ in 1758–1759. He was appointed to membership of the Académie Française in 1754 and quickly became second to Voltaire in the group for philosophes. In 1772 he became permanent secretary of this academy (but not that for sciences). He died of gall-stones on 29 October 1783” (Crépel, p. 160). En Français dans le texte 147; Norman 31; Parkinson Breakthroughs, p. 159; PMM 195; Roberts and Trent, p. 7; Roller and Goodman I, p. 26. Crépel, ‘Jean le Rond d’Alembert, Traité de dynamique,’ Chapter 11 in Landmark Writings in Western Mathematics 1640-1940 (Grattan-Guinness, ed.), 2005.
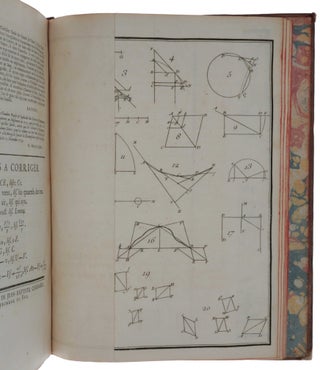
4to (219 x 165 mm) pp. [iv], xxvi, [ii], 186, [2], with engraved vignette on title and four folding engraved plates (some light browning). Contemporary calf, spine richly gilt in compartments (corners slightly worn).
Item #5878
Price: $4,500.00